AE00 - Rectangles
Byteman has a collection of N squares with side 1. How many different rectangles can he form using these squares?
Two rectangles are considered different if none of them can be rotated and moved to obtain the second one. During rectangle construction, Byteman can neither deform the squares nor put any squares upon any other ones.
Input
The first and only line of the standard input contains one integer N (1 ≤ N ≤ 10000).
Output
The first and only line of the standard output should contain a single integer equal to the number of different rectangles that Byteman can form using his squares.
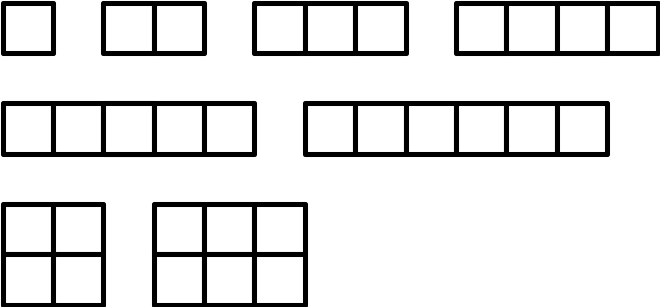
Example
For the input data:
6
the correct result is:
8
Task author: Jakub Radoszewski.
hide comments
|
|
Raginee:
2012-09-28 19:30:07
cant get the question any one explain plz.. |
|
|
Sumit Khanna:
2012-07-26 19:08:26
"1" appears to be the input for case 9,was getting WA before i ment my code to output 1 for 1 |
|
|
Teddy Budiono Hermawan:
2012-06-02 01:04:52
Here's the disappeared image http://main.edu.pl/images/PA2009/pro1.gif |
|
|
legrand:
2012-04-20 08:15:34
the picture has disapeared, can you fix it |
|
|
Sharavana guru:
2012-04-03 09:21:48
@ B.R.ARVIND thanks for the logic Last edit: 2012-04-03 18:50:34 |
|
|
B.R.ARVIND:
2012-03-28 19:49:42
for(int i=1;i<=n;i++) for(int j=1;i*j<=n;j++)s.insert(pair<int,int> (min(i,j),max(i,j))); tats the logic
|
|
|
amit kumar:
2012-03-04 18:06:16
square is also rectangle
|
|
|
Surya kiran:
2012-02-15 13:59:12
cant get the question any one explain plz..
|
|
|
giorgi:
2011-12-25 07:44:27
what is test 9
|
|
|
Rajkiran Rajkumar:
2011-12-11 04:46:07
@Jack Ibarra : Isn't it supposed to be 6? |
| Added by: | Race with time |
| Date: | 2009-05-03 |
| Time limit: | 1s |
| Source limit: | 50000B |
| Memory limit: | 1536MB |
| Cluster: | Cube (Intel G860) |
| Languages: | All except: ERL JS-RHINO NODEJS PERL6 VB.NET |
| Resource: | Algorithmic Engagements 2009 |

 RSS
RSS